Visualize a Wave Function
Can you visualize a normalized wave function on spacetime?
Let’s try with a simple example. The role of a wave function is to assign a complex number to each point (x, t) in spacetime. This is central to a quantum description of the world. The complex number at each point is interpreted as an amplitude, which determines a probability — the probability of measuring some physical quantity (like position or momentum) at that point.
But in the end, it’s just a complex number, of unit length.
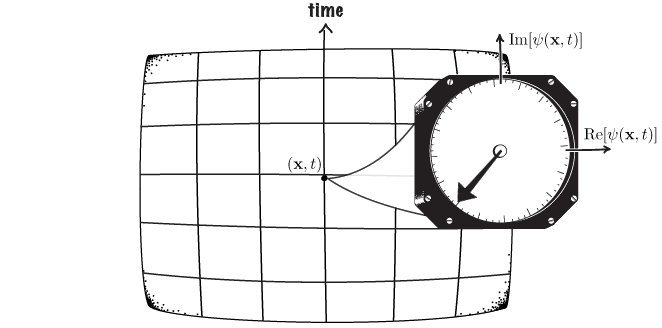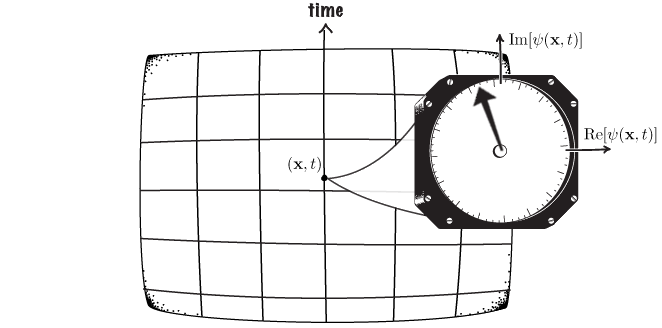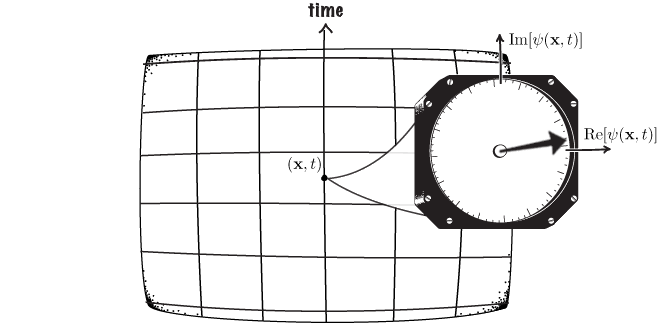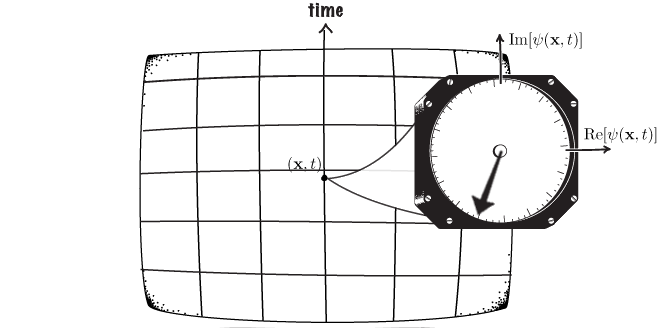
Now, a complex number lives on the complex plane — a plane with the vertical axis representing a complex value, and the horizontal axis representing a real value. And the complex numbers of unit length live on a circle around the origin. So you can think of these numbers as readings on a circular meter — like a speedometer or an altimeter — except that the meter reads amplitudes instead of speeds or altitudes.
That means you can visualize the wave function ψ(x, t) as assigning a meter-reading to each point in spacetime. And if I fix a point in space — like a spot on my kitchen floor — then I can trace through the history of this wave function over time. The result will be a smoothly changing meter reading. For example, the meter arrow might just spin around clockwise over time.
Then it would look something like the following.
Challenge Question: How would you characterize the “time-reverse” of this description of the world? Tune in next post for a discussion…
Edit: The above account is not quite right — see the post comments for more.
Soul Physics is authored by Bryan W. Roberts. Thanks for subscribing.
Want more Soul Physics? Try the Soul Physics Tweet.
Big problem – the overall wave function has unit length, but the wave function at any given point does not. You couldn’t normalize any wave function properly if your description were accurate – at best you could “normalize to the delta function.” psi*psi is the probability density and also the length square of the complex number you claim is unity. Some examples of real world wave functions which fail your description:
hydrogen atom,
harmoic oscillator,
bound states of the various square wells,
etc.
I think you’re confusing the path integral description of what you add up with the final wave function.
Thanks — I was a bit too quick with this idea.
I believe it would have been better to say that the contribution of histories to amplitudes can be thought of in my way: a path contributes exp(iS) to the total amplitude of an event (S=action along the path). And exp(iS) is just a reading on my meter.
But for where I’m going with this next time (visualizing time reversal), there’s a more informative way to fix up my idea. Just take little finite regions instead of points. And think of ψ as assigning to each little region a complex number properly inside the unit circle — i.e., in the picture, the arrow on the dial can move in and out as well as around, never touching the edge of the circle. Then you can normalize, and recover the usual description of the wave function. And then you can describe useful things like hydrogen atoms and harmonic oscillators that no one wants to do without, too.
I’ll make a note of this in the post text. Thanks again.
Except that the wave function can have magnitude greater than 1 at a given point, as long as that region is small enough. So it can go anywhere with respect to the dial.